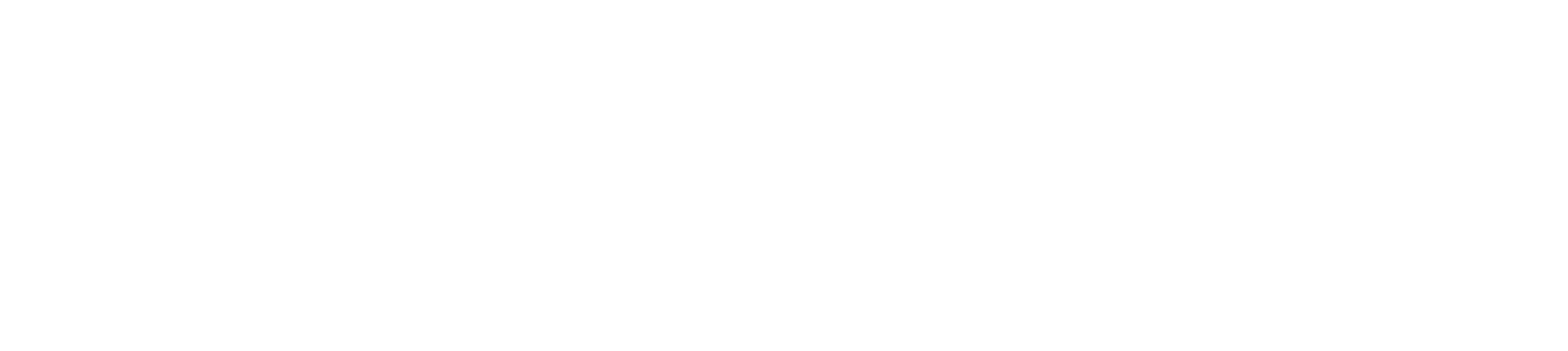Periodic boundary condictions

Many materials, such as woven and braided composites consist of repeating units at a meso-scale. Creating geometrically-accurate numerical models of these repeating units (unit cells) is complicated. However, these unit cells have symmetries that, if exploited, can reduce the work involved in building these models and can lead to more efficient numerical solutions. We have developed a mathematical framework for obtaining exact periodic boundary conditions that explore these symmetries.For grapheme structures analysed at the nano-scale, where the physics are non-linear, we have developed non-linear versions of the periodic boundary conditions.

Sample publications:

De Carvalho NV, Pinho ST, Robinson P, 2011, Reducing the domain in the mechanical analysis of periodic structures, with application to woven composites, COMPOSITES SCIENCE AND TECHNOLOGY, Vol: 71, Pages: 969-979

De Carvalho NV, Pinho ST, Robinson P, 2012, Numerical modelling of woven composites: Biaxial loading, COMPOSITES PART A-APPLIED SCIENCE AND MANUFACTURING, Vol: 43, Pages: 1326-1337

Gigliotti L, Pinho ST, 2015, Exploiting symmetries in solid-to-shell homogenization, with application to periodic pin-reinforced sandwich structures, Composite Structures, Vol: 132, Pages: 995-1005

Wehrkamp-Richter T, Carvalho N, Pinho ST, 2018, A meso-scale simulation framework for predicting the mechanical response of triaxial braided composites, Composites Part A: Applied Science and Manufacturing, Vol: 107, Pages: 489-506

Wehrkamp-Richter T, Vieira De Carvalho N, Pinho ST, 2018, Predicting the non-linear mechanical response of triaxial braided composites, Composites Part A: Applied Science and Manufacturing, Vol: 114, Pages: 117-135

Molecular Dynamics - Finite Element Method

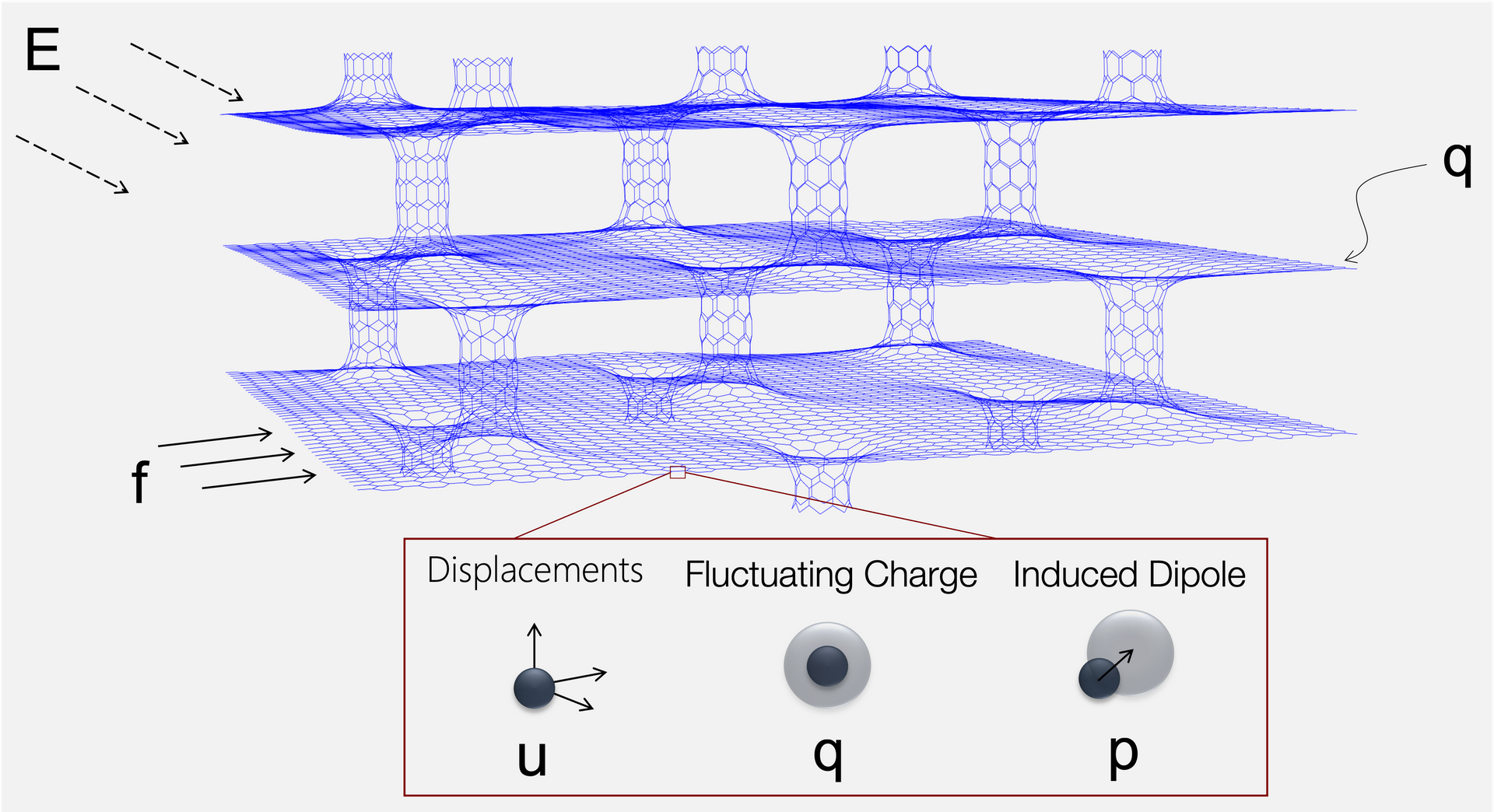
We coded a new Molecular Dynamics Finite Element Method (MDFEM) with a coupled mechanical-charge/dipole formulation. This MDFEM is equivalent to MD, as demonstrated by examples of fracture in carbon nanotubes (CNT), and electric charge distribution in graphene, but at a considerably reduced computational cost. It finds potential applications in design of nano-structures for desired electro-mechanical properties (e.g. stiffness, toughness and electric field induced vibrational/electron-emission properties).

Sample publications:

Wilmes, A. A. R., and Pinho, S. T. (2014), A coupled mechanical-charge/dipole molecular dynamics finite element method, with multi-scale applications to the design of graphene nano-devices, Int. J. Numer. Meth. Engng, 100; pages 243– 276

Mesh Superposition Technique

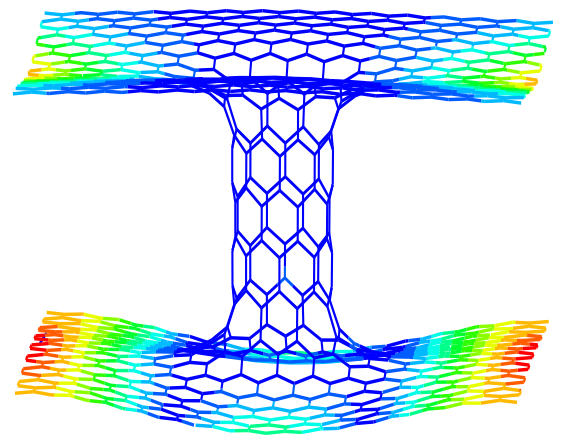
This Mesh Superposition Technique (MST) allows the progressive transition between differently-discretized subdomains. The interfaces between these subdomains are replaced by transition regions where the corresponding meshes are superposed. The use of MST eliminates stress disturbances at the interface between differently-discretized subdomains.

Sample publications:

L. Gigliotti, S.T. Pinho. Multiple length/time-scale simulation of localized damage in composite structures using a Mesh Superposition Technique,
Composite Structures. Volume 121, 2015, Pages 395-405

Luigi Gigliotti and Silvestre T. Pinho. Virtual Testing of Large Composite Structures: A Multiple Length/Time-Scale Framework. Journal of Multiscale modelling. Vol. 06, No. 03, 1550008 (2015)

Floating Node Method

The floating Node Method enables the modelling of discontinuities in a finite element. It can represent multiple intersecting discontinuities in one element. It does not require partial domain integration for partitioned elements.It can model both matrix cracking and delamination within the same element. It can predict matrix cracking/delamination interactions accurately.

Sample publications:

B.Y. Chen, S.T. Pinho, N.V. De Carvalho, P.M. Baiz, T.E. Tay, A floating node method for the modelling of discontinuities in composites, Engineering Fracture Mechanics, Volume 127, 2014, Pages 104-134

N.V. De Carvalho, B.Y. Chen, S.T. Pinho, J.G. Ratcliffe, P.M. Baiz, T.E. Tay, Modeling delamination migration in cross-ply tape laminates, Composites Part A: Applied Science and Manufacturing, Volume 71, 2015, Pages 192-203

B.Y. Chen, T.E. Tay, S.T. Pinho, V.B.C. Tan, Modelling the tensile failure of composites with the floating node method, Computer Methods in Applied Mechanics and Engineering, Volume 308, 2016, Pages 414-442

B.Y. Chen, T.E. Tay, S.T. Pinho, V.B.C. Tan, Modelling delamination migration in angle-ply laminates, Composites Science and Technology, Volume 142, 2017, Pages 145-155

Polymorphic Elements

Polymorphic enable multiscale analyses with an element-level management of coupling between scales. The location and extent of high-fidelity scale can evolve during analysis. With VCCT and CZM within their formulation, they enable natural multi-scale failure analysis of composite structures.

Sample publications:

E.S. Kocaman, B.Y. Chen, S.T. Pinho, A polymorphic element formulation towards multiscale modelling of composite structures, Computer Methods in Applied Mechanics and Engineering, Volume 346, 2019, Pages 359-387

Floating Connectors

This floating connector element allows adaptive positioning of hierarchical levels in a structural design. It provides combined mechanical/optimisation capability at element level. Floating connector elements can be inserted into a numerical model without modifying the individual components. They provide the ability to introduce a debond between the levels and use VCCT to optimise the position of a stiffener runout taking into account manufacturing defects.

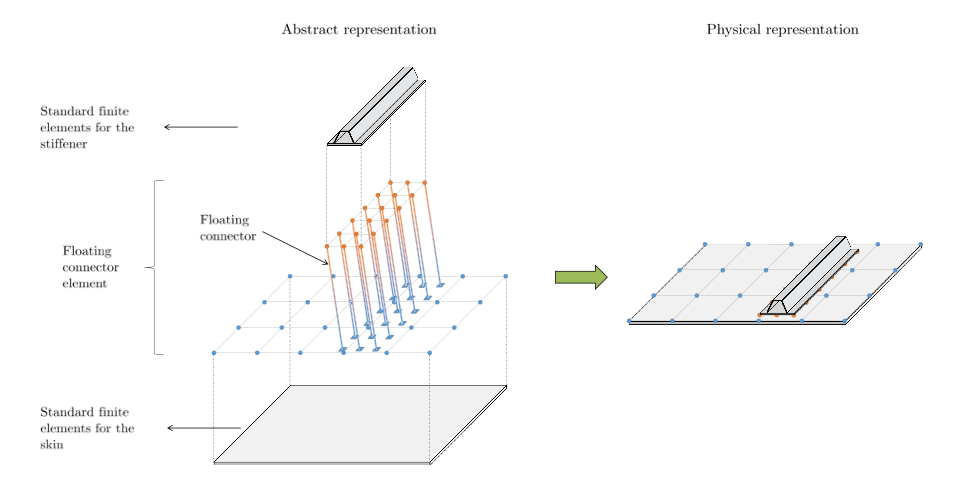
Sample publications:

E.S. Kocaman, B.Y. Chen, S.T. Pinho, A floating connector element formulation for multi-level modelling of composite structures, Composite Structures, Volume 251, 2020, 112532

Topology Optimisation

Evolving boundaries are an intrinsic part of many physical processes and numerical methods. We propose a novel formulation capable of explicitly discretising moving boundaries in an accurate and numerically-efficient way. It couples the floating node method (FNM) with LSM for the first time. The potential of this formulation is showcased within topology optimisation, showing greater geometrical accuracy and improvements in the optimum solution attained when compared to implicit methods.

Sample publications:

R.O.S.S. da Costa, S.T. Pinho, A novel formulation for the explicit discretisation of evolving boundaries with application to topology optimisation, Computer Methods in Applied Mechanics and Engineering, Volume 367, 2020, 113077